Rocket Plume Heat Transfer
Thermal Desktop® is commonly used for thermal analyses of spacecraft and propulsion systems. Less frequently, these tools are used for calculating the temperatures in supersonic exhaust nozzles, such as those in rockets or thrusters.
The temperature of the nozzle wall is an important aspect of rocket design. The exhaust-gas temperature typically exceeds the maximum allowable temperature of the nozzle wall material. The ability to estimate the wall temperature allows the design of a cooling system.
Four types of cooling systems can be modeled in Thermal Desktop: heat sink; thermal radiation; even regenerative (using FloCAD®). A difficult part of modeling the cooling system is approximating the heat transfer from the plume to the nozzle wall. The convective film coefficient can be estimated through a number of methods (Bartz equation, TDK boundary layer technique, etc); the coefficient is highly dependent on the axial location within the nozzle.
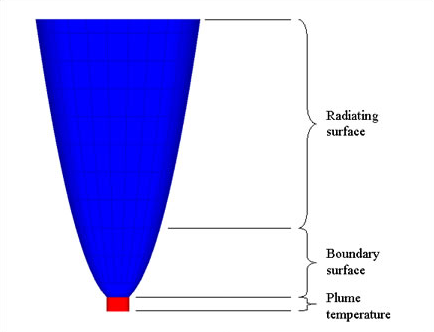
C&R Thermal Desktop® Model of a Radiating Nozzle
The steady-state solution is presented below. Note the increased temperatures on the front right side of the nozzle caused by variation in the heat transfer coefficient, called streaking. The radiating section of the nozzle shows varying wall temperatures as a result of the changing heat transfer coefficient.
When compared to the actual system, the convective heat fluxes for the radiating portion of the nozzle are underestimated by about 20%. These are reasonable results based on simplification of the geometry (no structural reinforcements were included) and assumptions made within the fluid properties and equilibrium reactions. The correction factor mentioned above could be adjusted to remove this error or provide a safety factor: a key benefit of model parameterization.
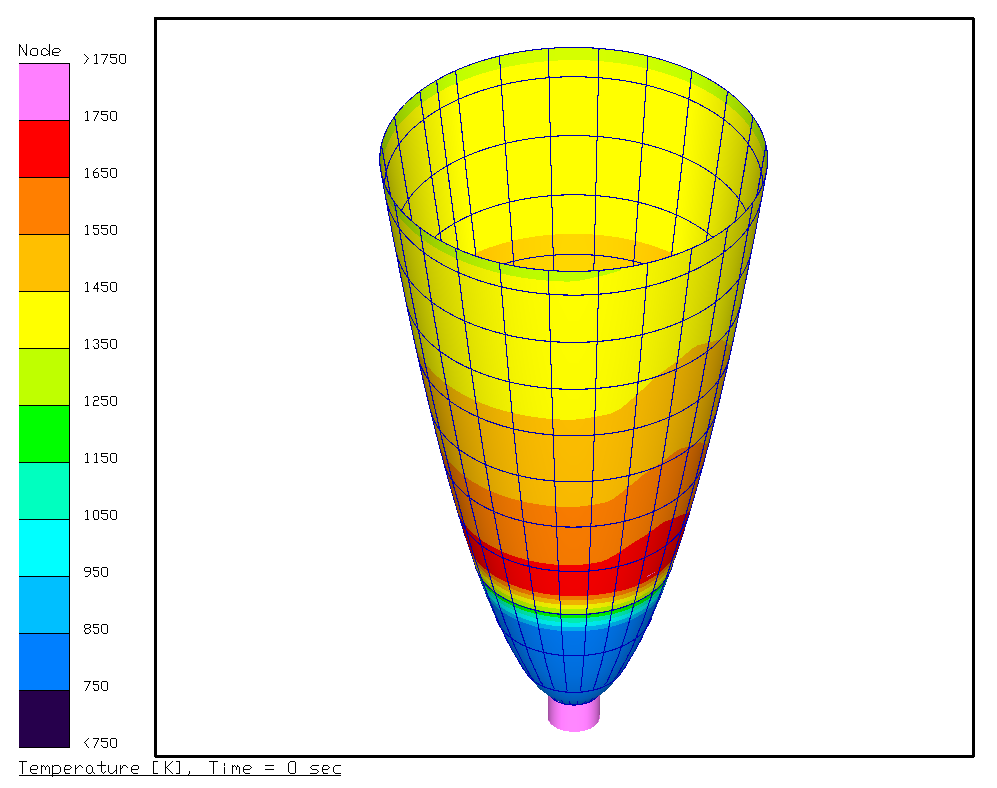
Steady-State Results of Plume Convection in a Radiating Nozzle Using the Bartz Equation with Streaking
Expansions of the model could be:
- Adding regenerative cooling in place of the fixed-temperature boundary condition
- Adding surfaces representing the throat (a torus, perhaps) and the combustion chamber
- Adding material properties to the inner wall of the nozzle or throat to evaluate recession of the material
- Adding a second, concentric surface around the nozzle and mapping solid elements between the surfaces to form a heat sink nozzle
- Mapping the results to a NASTRAN or ANSYS structural FE model
